10.1: Infinite Sequences and Summation Notaion

A sequence is a function whose domain is all natural numbers.

 represents the first time of the sequence, while  denotes a positive integer, or the nth term.
Sequences often appear as  , representing the first three terms of the sequence, as well as the nth term.

An infinite sequence is a function whose domain is the set of positive integers.

For example: Find the eighth term of the sequence,  with the first four terms: 1/3, 1/2, 3/5, 2/3...
Eighth term:
 n=8
= 4/5

There are two basic forms of writing sequences, recursive  and explicit.


Recursive Form






On the other hand, the same sequence may be represented in this way as well:

Explicit Form




Summation Notation
Summation notation is another alternative form of a sequence that can be used to find the sum of multiple terms of an infinite sequence.

Summation Notation
 

k represents the lower limit, while m represents the upper limit.
k also represents the index of summation.

This picture also re-explains summation notation in slightly different terms.


There are also two rules that apply to the sums of a constant.

Theorems on the Sum of a Constant:

1) (c being a constant)


2)


There are also a few theorems that derive from the following original theorem on sums.

Theorem on Sums


Addition

Subtraction


Constants
 for every real number c


To evaluate a sum:

Find the sum:



To find the sum, substitute the integers 1 (lower limit) through 3 (upper limit) in for k and add the resulting terms together.

(1+1) + (2+1) + (3+1)= 9

That's it for now, thank you!

Julia Wilkins

9.5 - Systems of Linear Equations in More Than Two Variables (Matrices)

A Matrix is a rectangular array of numbers, symbols, or expressions. 


The rows of a matrix are the numbers that appear horizontally, and the columns of a matrix are the numbers that appear vertically next to each other.

The matrix that is obtained from a system of linear equations is called the matrix of a system.

For example:  

turns into the matrix of:

 

where the numbers in the matrix (elements) are the coefficients of the system of linear equations in the previous set of equations.  These kinds of matrices are called augmented matrices because the matrix of the system can be obtained from the coefficient matrix.

Theorem on Matrix Row Transformations:

          Given a matrix of a system of linear equations, a matrix of an equivalent system results if :

                 1) two rows are interchanged

                 2) a row is multiplied or divided by a non zero constant

                 3) a constant multiple of one row is added to another row

Using matrices to solve a system of linear equations 

turns into the matrix:

Now, once you have 0's under both of the first numbers for the 2nd and 3rd rows, you go to the second row and multiply it by the number that will make the 1st non-zero number equal to 1.  In this case you multiply the row by 1/5 to make 5 equal to 1.

This is called the echelon form of a matrix.  From this, you get that:

1z=2 so z=2

and you can plug that into the original equations to solve for x and y using substitution.  

Another way to solve a matrix problem is to plug the original coefficient matrix into a calculator and go to the math matrix menu and get the reduced row echelon form of the matrix, giving you the 3 solutions. (x,y,z)

The solutions found to this equation after going back and solving by substitution are x=4, y=3, and z=2

Echelon form of a matrix:

  1) the first nonzero number in each row is 1

  2) the column containing the first nonzero number in any row is to the left of the column containing the first nonzero number in the column below

  3) rows consisting entirely of 0's may appear at the bottom of the matrix, but no where else

Guidelines for finding the echelon form of a matrix:

  1) locate the 1st column that contains nonzero numbers, and apply transformations so that the number 1 is in the first row of that column

 

  2) apply row transformations of the type  above for j>1 to get 0 under the number 1 obtained in step 1 for each remaining row.

   

  3) disregard the first row. Locate the next column with nonzero elements, and apply transformations to get the number 1 into the second row of that column.

 4) apply row transformations of the type above for j>2 to get 0 under the number 1 obtained in step 3 of this process for each remaining row.

5) disregard the first and second rows.  Locate the next column that has nonzero elements and repeat the procedure again.

6) continue this process until the echelon form is completed.

That's about all you need to know about matrices, although the easiest way to do them is just by plugging the matrix into a calculator to find the reduced row echelon form.  Here are a couple useful links in helping to solve matrices.

http://www.wyzant.com/Help/Math/Precalculus/Matrices/Reduction_to_Row_Echelon_Form.aspx

http://www.youtube.com/watch?v=pyUEsefV4uY

Thanks, 

Michael Levitsky

Chapter 4 Review

Alright guys, I have been assigned to review extra exciting chapter 4. Here goes...

Okay so 4.1 was all about polynomial functions of a degree greater than 2.
We are aware that...


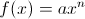
If n=1, the graph of f will be a line through the origin.  If n=2, the graph is a parabola with the vertex at the origin.  If n=3 the graph of the polynomial flattens out around the origin
                          before passing through.

-If n is an odd positive integer, then f is an odd function and the graph of f is symmetric with respect to the origin.
-If n is an even positive integer, the f is an even function and the graph of f is symmetric with respect to the y-axis.   

-As the exponent (n) increases, the graph becomes flatter at the origin. 
The graph will also rise more rapidly for x >1.

-Turning points for a graph of a polynomial are the high and low points of the graphed polynomial. An n-degree polynomial has at most n - 1 turning points. 

In 4.2, we learned the properties of division.

One form of division is titled long division.  This name is by no means an exaggeration. 

Divide x²+3x+1 by x⁴-16

X⁴-16 is not divisible by x²+3x+1, but by long division we can find a quotient and a remainder.

Here it is….            

                                    x²- 3x +8                           Quotient

x²+3x+1   x⁴+0x³+0x²+0x+16                               

                  x⁴+3x³+x²                                                         

                       -3x³-x^{2                                                           subtract}

                        -3x³-9x²-3x                                   

                                   8x²+3x-16                           subtract

                                  8x²+24x+8

                                        -21x-24                          Remainder

Remainder Theorem: If a polynomial f(x) is divided by x - c, then the remainder is f(c).

Factor Theorem: A polynomial f(x) has a factor x - c if and only if f(c) = 0.

In section 4.3, we learned about the zeros of polynomials.

Fundamental theorem of Algebra: if a polynomial f(x) has positive degree and complex coefficients, then f(x) has at least one complex zero.

Theorem on the Maximum Number of Zeros of a polynomial: A polynomial of degree n > 0 has at most n different complex zeros.

Zeros of multiplicity: If a factor x - c occurs m times in the factorization of the polynomial, the c is a zero of multiplicity m of the equation f(x) = 0. 

                - the zero of multiplicity is how many times x - c occurs in the factorization

•     If m is an even number, both ends of the graph of the polynomial point in the same direction
•     If m is an odd number, the two ends of the graph point in opposite directions 

Number of zeros of polynomials: if f(x) has degree n > 0 and if a zero of multiplicity m is counted m times, then f(x) has exactly n zeros.

Descartes' Rule of Signs: there are two parts to this one....

1. The number of positive real zeros of f(x) either is equal to the number of variations of sign in f(x) or is less than that number by an even integer.

2. The number of negative real zeros of f(x) either is equal to the number of variations of sighn in f (-x) or is less than that number by an even integer.

so, for example, if f(x) has three changes in sign, the polynomial either has three positive zeros or one positive zero. Furthermore, if f (-x) only has one change in sign, there is only one negative zero of the polynomial. 

Section 4.4 is about complex and rational zeros of polynomials.

Rule on Conjugate Pair Zeros of a Polynomial: if f(x) has a degree greater than 1 with real coefficients, then...

if z = a+bi (b not equal to zero) is a complex zero, then z = -bi is also a zero of f(x).

Express x⁵-4x³+x²-4 in linear and quadratic polynomials with real coefficients that are irreducible over Ɍ:

= (x⁵-4x³) + (x²-4)                             group terms

= X³(x²-4) + 1(x²-4)                          factor out x³

= (X³+1) + (x²-4)                               factor out x²-4

= (x+1) (x²-x+1) (x+2) (x-2)            factor as the sum of cubes and the difference of squares

and boom! there you have it.

Now, on to finding the possible rational solutions of an equation....

First, one must know that the possible rational zeros are:

    

   Factors of the constant term of the polynomial

Factors of the leading coefficient of the polynomial

     So when given a polynomial, to find the rational solutions of the equation, you find all the choices, positive and negative, for c/d (the numerator divided by the denominator). 

     By dividing synthetically with one of the zeros you obtained, you should see if when divided, the remainder if zero.  If it is, that factor is a rational solution of the equation. 

    Keep dividing by the choices of c/d until you have gone through all of them. Remember, if a factor does come to zero, use the new polynomial formed when continuing synthetic dividing. 

    

Finally, on to 4.5. This section is all about rational functions.

A function is rational if:

 

, where g(x) and h(x) are polynomials.  The domain of f consists of all real numbers except the zeros of the denominator h(x).

Definition of a vertical asymptote: The line x = a is a vertical asymptote for the graph of a function if

                                           as x approaches a from either the left or the right.

Definition of a horizontal asymptote: The line y = c is a horizontal asymptote for the graph of f if:

f(x) approaches c as x approaches infinity or negative infinity.

Guidelines for Sketching the Graph of a Rational Function

1. Find the x-intercepts (the real zeros of the numerator g(x) ) and plot the points on the x-axis

2. Find the real zeros of the denominator h(x).  For each real zero a, sketch the vertical asymptote x = a with dashes.

3. Find the y-intercept f (0), if it exists, and plot the point (0, f (0) ) on the y-axis.

4. If there is a horizontal asymptote x = c, sketch it with dashes.

5. Sketch the graph of f in each of the regions on the xy plane determined by the vertical asymptotes.

And there you have it folks; chapter 4 in a nutshell. Hope it helps

Chapter 2 Review

Chapter 2 Review

So here's a basic summary of what we learned in Chapter 2- Equations and Inequalities

-An Equation is a statement that 2 quatities or expressions are equal

-A linear equation is an equation that is written in the form ax+b=0 where a does not equal 0.

Solving a Linear equation








-Simple Interest Formula
I=Prt where I is the simple interest, P is the principal amount, r is the interest rate, and t is time.


-Zero Factor Theorem



-Special Quadratic Equation



-Completing the Square- There are 2 ways to complete the square, depending on on the sign of the equation.

If the sign is positive, the equation is:


If the sign is negative, the equation is:


-Quadratic Formula- The quadratic formula is used when an equation is unable to be factored easily, so you use this formula to get the value(s) of x.



-Properties of i



Conjugate of a Complex number: If.... (z=a+bi) then its conjugate is...a-bi

ex. Complex #- 8+4i
Conjugate- 8-4i

-Solving an equation using grouping




-Inequalities: an inequality is a statement that 2 quatities or expressions are not equal.

Solving an inequality



-Properties of Absolute Values




ex.






-Solving a Quadratic Inequality


Then, use the values of x on a number line and plug in numbers (I used -5, 0, and 5) in the different intervals to find if that interval is positive or negative.

So the answer would be:


Well that's about all we did in Chapter 2. Good luck on the final.

-Michael Levitsky